
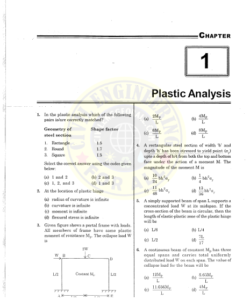
Table of Contents
IES MASTER GATE MATERIAL
PLASTIC ANALYSIS (STEEL STRUCTURES)
GATE – PSU – IES – GOVT EXAMS – STUDY MATERIAL
FREE DOWNLOAD PDF


CONTENTS
- Plastic Analysis – Objective Questions and Solutions
- Plastic Analysis – Conventional Questions and Solutions
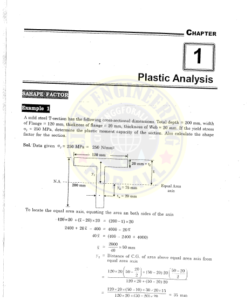
Plastic analysis
In plastic analysis and design of a structure, the ultimate load of the structure as a whole is regarded as the design criterion. The term plastic has occurred due to the fact that the ultimate load is found from the strength of steel in the plastic range. This method is rapid and provides a rational approach for the analysis of the structure. It also provides striking economy as regards the weight of steel since the sections required by this method are smaller in size than those required by the method of elastic analysis. Plastic analysis and design has its main application in the analysis and design of statically indeterminate framed structures.
Theorems of Plasticity
There are three basic theorems of plasticity from which manual methods for collapse load calculations can be developed. Although attempts have been made to generalize these methods by computers the calculations based on these methods are still largely performed manually. The basic theorems of plasticity are kinematic, static, and uniqueness, which are outlined next.
Kinematic Theorem (Upper Bound Theorem)
This theorem states that the collapse load or load factor obtained for a structure that satisfies all the conditions of yield and collapse mechanism is either greater than or equal to the true collapse load. The true collapse load can be found by choosing the smallest value of collapse loads obtained from all possible cases of collapse mechanisms for the structure. The method derived from this theorem is based on the balance of external work and internal work for a particular collapse
mechanism. It is usually referred to as the mechanism method.
Static Theorem (Lower Bound Theorem)
This theorem states that the collapse load obtained for a structure that satisfies all the conditions of static equilibrium and yield is either less than or equal to the true collapse load. In other words, the collapse load, calculated from a collapse mode other than the true one, can be described as conservative when the structure satisfies these conditions. The true collapse load can be found by choosing the largest value of the collapse loads obtained from all cases of possible yield
conditions in the structure. The yield conditions assumed in the structure do not necessarily lead to a collapse mechanism for the structure. The use of this theorem for calculating the collapse load of an indeterminate structure usually considers static equilibrium through a flexibility approach to produce free and reactant bending moment diagrams. It is usually referred to as the statical method.
Uniqueness Theorem
It is quite clear that if a structure satisfies the conditions of both static and kinematic theorems, the collapse load obtained must be true and unique. Therefore, the uniqueness theorem states that a true collapse load is obtained when the structure is under a distribution of bending moments that are in static equilibrium with the applied forces and no plastic moment capacity is exceeded at any cross section when a collapse mechanism is formed. In other words, a unique collapse load is
obtained when the three conditions of static equilibrium, yield, and collapse mechanism are met. It should be noted that an incremental elasto-plastic analysis such as that described satisfies all three of these conditions:
(1) static equilibrium—elastic analysis is based on solving a set of equilibrium equations contained in matrices;
(2) yield—the moment capacity for every section is checked and a plastic hinge is inserted if plastic moment is reached in any section; insertion of a plastic hinge in the analysis ensures that the moment capacity is not exceeded; and
(3) mechanism—the formation of a collapse mechanism is checked by (a) determining whether the determinant of the stiffness matrix is zero; a zero value leads to an error message if a computer is used for analysis; and (b) excessive deflections if an exact zero stiffness cannot be detected. Hence, the collapse load obtained from an elastoplastic analysis is, in general, unique.
Uniformly Distributed Loads (UDL)
When using the mechanism method, the main difficulty in dealing with a distributed load is to calculate the external work as it normally requires integration for its evaluation. However, some convenient concepts can be developed to circumvent this difficulty. The following example demonstrates the treatment of uniformly distributed
loads using both statical and mechanism methods.
Continuous Beams and Frames
In order to examine all possible collapse modes for continuous beams and frames, the concept of partial and complete collapse is introduced in the following section. In particular, partial collapse often occurs in continuous beams and frames upon which multiple loads are applied.
Partial and Complete Collapse
The discussion in the previous sections focused mainly on simple indeterminate structures. Typically, these structures have n degrees of indeterminacy and require n + 1 number of plastic hinges to form a collapse mechanism. In such cases, the structures are said to have failed by complete collapse. We define complete collapse as When a structure with n degrees of indeterminacy collapses due to the formation of p number of plastic hinges where p ¼ n + 1, the structure fails by complete collapse; in this case, determination of the member forces for the whole structure at collapse is always possible. However, partial collapse of a structure can be defined as When a structure with n degrees of indeterminacy collapses due to the formation of p number of plastic hinges where p < n þ 1, the structure fails by partial collapse; in this case, it may not be possible to determine the member forces for some parts of the structure. Structures may fail plastically by complete or partial collapse. In either case, the stiffness of the structure at collapse is zero. For continuous beams and frames where the degree of indeterminacy is large, partial collapse is not uncommon.
Stiffness Method
Contrary to the traditional thinking that plastic analysis is performed either by simple manual methods for simple structures or by sophisticated computer programs written for more general applications, this book intends to introduce general plastic analysis methods, which take advantage of the availability of modern computational tools, such as linear elastic analysis programs and spreadsheet applications. These computational tools are in routine use in most engineering design offices nowadays. The powerful number-crunching capability of these tools enables plastic analysis and design to be performed for structures of virtually any size. The amount of computation required for structural analysis is largely dependent on the degree of statistical indeterminacy of the structure. For determinate structures, use of equilibrium conditions alone will enable the reactions and internal forces to be determined. For indeterminate structures, internal forces are calculated by considering both equilibrium and compatibility conditions, through which some methods of structural analysis suitable for computer applications have been developed. The use of these methods for analyzing indeterminate structures is usually not simple, and computers are often used for carrying out these analyses. Most structures in practice are statically indeterminate.
Structural analysis, whether linear or nonlinear, is mostly based on matrix formulations to handle the enormous amount of numerical data and computations. Matrix formulations are suitable for computer implementation and can be applied to two major methods of structural analysis: the flexibility (or force) method and the stiffness (or displacement) method.
The flexibility method is used to solve equilibrium and compatibility equations in which the reactions and member forces are formulated as unknown variables. In this method, the degree of statiscal indeterminacy needs to be determined first and a number of unknown forces are chosen and released so that the remaining structure, called the primary structure, becomes determinate. The primary structure under the externally applied loads is analyzed and its displacement is calculated. A unit value for each of the chosen released forces, called redundant forces, is then applied to the primary structure (without the externally applied loads) so that, from the force-displacement relationship, displacements of the structure are calculated. The structure with each of the redundant forces is called the redundant structure. The compatibility conditions based on the deformation between the primary structure and the redundant structures are used to set up a matrix equation from which the redundant forces can be solved.
The solution procedure for the force method requires selection of the redundant forces in the original indeterminate structure and the subsequent establishment of the matrix equation from the compatibility conditions. This procedure is not particularly suitable for computer programming and the force method is therefore usually used only for simple structures.
DOWNLOAD LINK : IES MASTER Plastic Analysis Objective and Conventional Questions and Solutions for GATE PSU IES GOVT EXAMS Free Download PDF www.CivilEnggForAll.com
DISCLAIMER : THIS WEBSITE IS NOT THE ORIGINAL PUBLISHER OF THIS BOOK ON NET. THIS E-BOOK HAS BEEN COLLECTED FROM OTHER SITES ON INTERNET. ALL THE RIGHTS ON THIS BOOK BELONGS TO IES MASTER ACADEMY

Leave a Reply